Projective Transformation relies on concatenations of elementary incidence.
Iterative Transformation
![]()
![]()
![]()
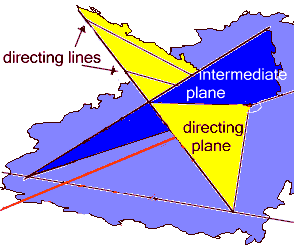
Here, planes project lines. The red line is rotated, by a gray plane, from the light-blue, base plane to the intermediate plane, and rotated from there by another gray plane back to the base plane in a fresh orientation.
For this to work, all the planes involved must have the same point in common.
(this page is still being Drafted)
As we have seen, Projective Geometry has no Axiom of Equality, and also no Axiom of Curvature. That is, there are no operations of elementary incidence leading to either.
But there are iterative operations of elementary incidence which can
- step a line in a plane around a point, like the red line stepping on the light blue plane, on the left,
- step a plane around a line, like the two gray planes stepping round the directing lines, on the left.
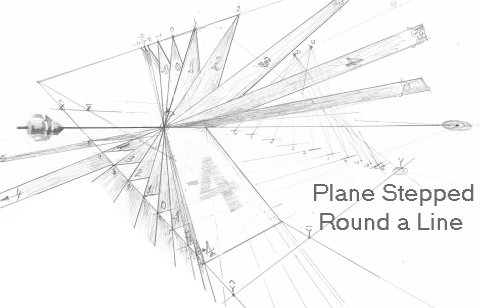
I show above a step-turning plane cutting two “fixed” planes, which cut each other in a “fixed” line (it's at the top of the picture). The turning plane cuts them in one line each, which turn as it turns.
If we think of the line on which the plane turns as an “axle”, or a “rotor”, with “bearings” (points) in the fixed planes, these turning lines turn in the bearings and in the fixed planes.
If two lines, skew to each other, are drawn in the “fixed” planes, one to each plane, the turning plane's lines will cut them in one point each, which will step with the turning plane.
The join of these two stepping points in the skews is said to be a “generator”. Now each generator, as it is made, comes out skew to every other, so it is not possible for a plane to be common to any two of them, no matter how “closely” they approach each other.
(Recall that, in any case, in this raw projective context, ‘closeness’ is meaningless).
The generators therefore cannot form the planar “infinitesimals” required for a continuous, differentiable surface, so form no surface at all—but the central projections of these generators into a fourth plane are step-wise tangent to, and therefore form, a differentiable curve. It is a conic, and the first curve to arise from elementary incidence.
There is a subtle but tenacious error associated with this. The “generator” is so-called because it is held to generate a continuous surface, namely a hyperboloid.
But such a surface, to be continuous, should be differentiable, and we have just noted above that mutually skew lines cannot form a differentiable surface. Why do we think they can?
I believe it is because it is popularly supposed that, “all the infinitely-close points in the moving skews work together to draw out the surfaces, as infinitely-many, infinitely-crowded, parallel conics.”
But this does not and cannot happen, because lines are not composed of points so there are no points available to trace out conical locii. And, even if there were such points, they could not keep rigid station with each other, and could not in any event form parallel conics: they would be skew to each other.