Home         Site Map         Tutorial Material
 

Notes on Directivity

    
  The direction of a line is generally expressed as an angle to some reference line, because, by itself, a line does not have a direction.  So an angle is an interval. This angle can range between 0 and 360°, or 2π radians, but, in ordinary usage, it does not in fact measure direction: instead, it measures heading.  (However, direction increases anticlockwise, while heading increases clockwise, and they have zeroes 90° apart – but this is just by convention, not principle.)

If, for the moment, we let the “Euclideo-Newtonian Dogma” stand, which means that we will suppose that the accustomed way to calibrate the relative rotation of projective lines is proper, then, by that calibration, the most two such (co-planar) lines can differ in direction is 90°, or π/2 radians. Rotation of the line away from π/2, in either sense, clockwise or anticlockwise, simply has it re-approach the direction of the reference line.

Clearly, in these circumstances the simple pairing of angle and direction does not serve us very well, because while angle and heading may increase indefinitely, direction or orientation may not, so we should, perhaps, rather speak of alignment.

That is, we may say that two co-planar lines
  • are least aligned, or most misaligned, when they stand at a right angle to each other
  • and most aligned, or least misaligned, when they stand parallel to each other
 So we need a system that will assign a value of
  • 1.0 (one) to maximum alignment at 0.0°, and at integral multiples of 360.0°, or 2π radians,
  • 0.0 (zero) to maximum misalignment at odd integral multiples of 90.0°, or π/2 radians, and
  • 0.5 (half) to half alignment at odd integral multiples of 45.0°, or π/4 radians,
and assign corresponding values for intermediate alignments.

We find it in cos2φ, where φ is the angle between the line and the reference line, as in ordinary usage—namely, the line's heading.

The Directivity, or Alignment, A, of a line relative to a reference line is, very simply,

cos2φ.

I sometimes use the term, “Alignment”, for this quantity, as it perhaps better expresses what it measures – namely, how near two lines are to being aligned, or, ‘in line’.		    
 

When we come to consider the directivity of a pencil of N, co-planar lines (where N is a signless integer > |1|) with respect to some reference line also in the plane, but not in the pencil,

we sum the squares of the cosines of all N angles
made by the lines with the reference line,
and divide that sum by N.

In other words, we find the mean directivity of the lines in the pencil, and take this to be the directivity of the whole pencil. Note that this is an arithmetic mean, not a geometric or harmonic one.

Directivity of a pencil of N lines


 

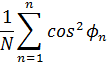
maths1

 

where φn is the angle to the reference line of line n of the pencil.

A ‘co-vertical’ pencil.

co-vert

Note also that, while the lines of the pencil must be co-planar, they need not be “co-vertical”. That is, they need not all pass through the same point on their plane. So we also consider here something that could be described as a ‘distributed’, or, ‘scattered’, pencil. This allows us, for example, to discover, should we wish to do so, the directivity of the lines-of-motion of all - or of just some - of the planets taken together.

A ‘distributed’, non-covertical pencil.

distributed
 

We next consider how to find that single line which has, with respect to the reference line, the same directivity as the pencil. This line has the orientation of the whole pencil, and the directivity of the pencil measures how ‘strongly’, or, ‘how well’, the pencil has this orientation. Let us call this line the bisectoid of the pencil.

Just as a centroid is not a centre, but is a point that comes as closely as it can to being equidistant from all the points in a scatter of points, and is thus centre-like, so a bisectoid is not a bisector, but is a line that comes as closely as it can to being equiangular with all the lines in a scatter of lines, and is thus bisector-like.

Let φ be the angle of a general line to the reference line. The pencil's directivity with respect to this line will be

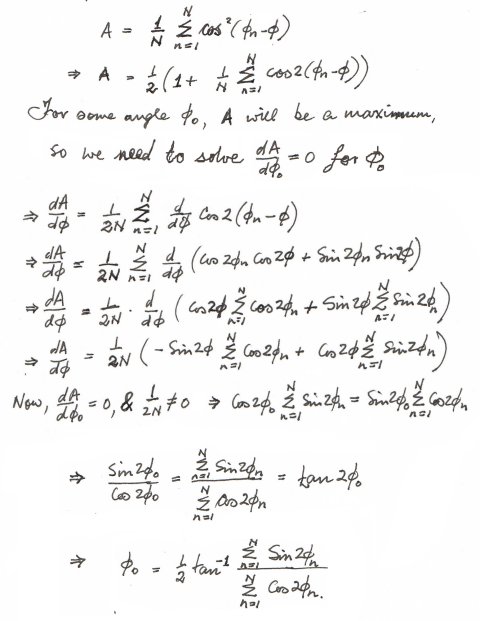
derivation.jpg

 Bisectoid of a pencil

 

math1

 
       
Home         Site Map         Tutorial Material;