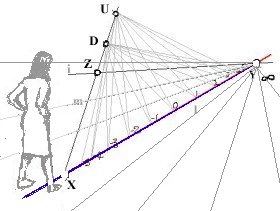
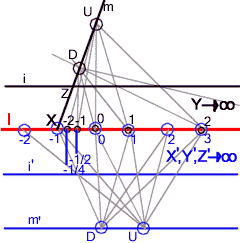
 The sketch on the left depicts two linear measures on the same (red) line, l. Let us call them the "black" and the blue". The sketch on the left depicts two linear measures on the same (red) line, l. Let us call them the "black" and the blue".
One of these, the blue,
is the Standard Measure, derived as in the animation above right.
The black is new. It has one of the vertices, X, of its (non-degenerate) triangle XYZ placed locally on line l - on the page, so to speak - and Y on l at the infinite. Accordingly, the
intermediate line i through Y and Z lies parallel to l.
Line m, the bearer of directing points U and D, passes through X and Z,
as always.
The (semi-degenerate) blue triangle X'Y'Z',
on the other hand, has all three of its vertices on l and all at the same place, namely infinity, so lines l, m' and i' (made distinct) are parallel.
We already know that the blue measure has "equal" intervals, even as "equal"
is ordinarily understood.
How is that with the black measure?
Well, as we have seen, it too, within its own
context, has equal intervals. Yet,with the exception of the intervals
between 0 and 1 (here deliberately made to match), correspondingly-numbered black and blue intervals
clearly differ.
Both measures were produced by stepping in identical ways:
the only difference between them concerns
the locations of vertices X and Z.
The black versions of these are local.
All others, black or blue, lie together in the same place on l, namely, at the infinite.
However, the black interval between 1 and 2 has been chosen to match the blue
interval between 1 and 3,
so by the blue measure, that black interval is two blue intervals in length. If
you look to the first black interval to the left of interval (0,1),
you will see that it has a length of half a blue interval, and then you will see
that the black interval to the left of that black interval has a length of one quarter of a blue interval - that is, half
of a half of a blue interval. It appears that if we step left with the black
intervals, we will find that each is half the size of the one before, as guaged against the blue measure. Conversely,
if we instead step right, successive black intervals will be found to double in length, again as guaged against the blue measure.
We have a constant common
ratio, or characteristic
multiplier, which is 2.0 going
right, and 0.5 going left. By the
blue measure, the black measure is a geometric
series, or geometric progression.
Now, this common ratio is a "nice" one, achieved by conscious construction: it
is either an integer, or that integer's reciprocal, perforce a nicely rational number. It will not always be so nice with other, less deliberate constructions,
but it will always be constant.
As we step left, the black intervals
diminish with respect to to the blue, and it is clear that no number of steps
will take the black measure past vertex X - but it is equally clear that an exactly infinite number of steps will bring the measure exactly to X. X is a limit. By the same reasoning, so is Y.
The black series, going
right, must end precisely at infinity, at Y (this may come as a surprise: the German
word for infinity is Unendlichkeit, a
'state of unending'. All the same, it is an ending-place for this measure).
On the other hand, the line l, bearing
these measures, does not stop at Y, as lines have no ends. Neither
does line l halt at X,
the other limit of this measure. This fetches us up sharply against the
realisation that some measures do not span the line in which they sit. The black measure is one. The blue is not.
The general lesson here is that, although no one measure
can be judged absolute, or superior to any other, any one may nevertheless be
taken as standard, and used to "measure" the others, just as we have done above.
It is a matter of convention. It is natural and convenient for us
to use the blue measure, and we conventionally do—and, though it is not
absolute, we are predisposed
to regard it as absolute.
|

 The sketch on the left depicts two linear measures on the same (red) line, l. Let us call them the "black" and the blue".
The sketch on the left depicts two linear measures on the same (red) line, l. Let us call them the "black" and the blue".