Line Moving Skew to Itself

A Study of Skewed Vectors

The view here is from ∞, through an infinitely-powerful telescope.

You may click and drag the tips and tails of the primary vectors, and control the rotation of the planes by dragging the thin blue, dash-dot line.
Temporarily, we abandon projective principle, and say that
the vectors, along with everything else in the construction,
obey the “Euclideo-Newtonian” dogma of naïve realism
(in which infinity, parallelity, circularity and orthogonality
are all considered absolute).

Note that the whereabouts of the turning points depends on the arbitrary selection of the component's planes (which, incidentally, need not be at right angles),
so they do not, therefore, have unambiguous, determinate locations—
unless the primary vectors are co-planar and parallel (i.e., not skew), when the two turning points degenerate into a unique, constant location, independent of component planes.

!! Please click 'n' drag above to test this assertion for yourself !!

Turning places must be determinate, but we see above that,
when the primary vectors are skew,
these places are indeterminate.

Without determinate points around which to turn, the line cannot turn.
—It follows immediately that a line cannot move skew to itself—

—Another approach—

We show two primary vectors, one red, one blue, initially set skew to each other, with each lying in one of two, parallel planes at right angles to our telescope. We take the blue vector and its plane to be “nearest” to our viewpoint.

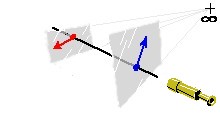
The vectors notionally indicate the motion of two points in the line that joins them and passes between the two planes.

We have a “skewed couple”,

so that, as a whole thing, the line would seem obliged to be moving skew to itself.

Since a line, even a Euclidean one, can move only by Rotation around a point and in a plane, we seek the point around which this line must rotate while it moves skew to itself.

Using Thales' Theorem, each vector is resolved into ‘orange’ and ‘black’ components in two planes at right angles - with one plane common to the orange components, the other common to the black - both in the connecting line, around which they may be rotated.

The point T around which the moving line is turning in a rotating plane is found by noting where the join of the tips of the vector components in the plane cuts the moving line.

So there appear to be two turning points, T_black and T_orange, (named for the corresponding components' colours). On the right, we show it to be just that—appearance.