Turning Points
Caveat.
Under the strictest application of geometric axioms,distances and vectors – and their equations – cease to work.
Here, we temporarily set aside strict geometric axioms,
and take the Euclideo-Newtonian dogma to apply instead.
Geometric elements in any event cannot be tugged about
like material objects—but here, for the moment,
we take it that they can.
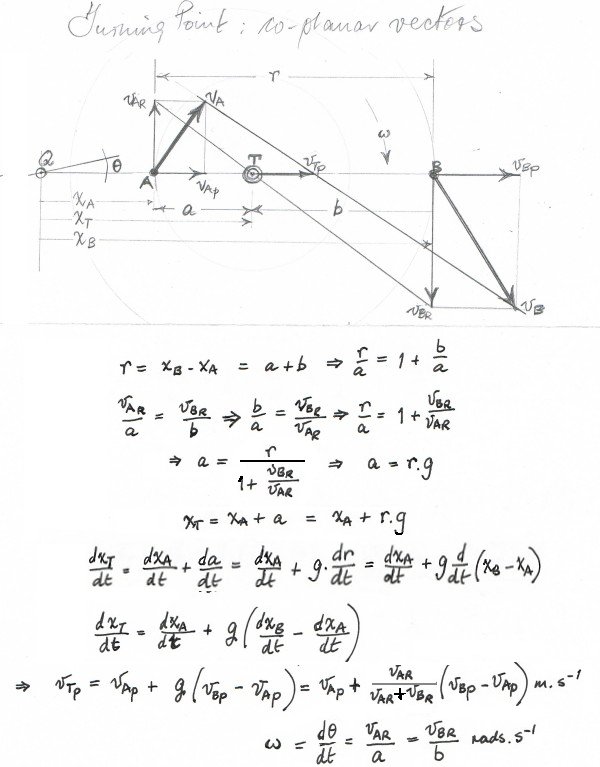
We have a turning line bearing two points, A and B, at distances xA and xB from a fixed, arbitrary point, Q, also on the line. The motions of A and B are described by co-planar vectors, vA and vB, respectively. These motions are resolved into components, vAr and vBr, at right angles to the turning line, and components, vAp and vBp, parallel to (actually in) the line.
Point, T, around which the line is turning as a result of the co-planar motions of the points A and B, is found at the place on the turning line at which the join of the vector-tips of the components at right angles to the line, vAr and vBr, cuts that line.
The velocity of T has the same orientation as the turning line, and its vector, vTp, lies between T, and the place on the turning line at which the join of the tips of the original, unresolved vectors, vA and vB, cuts that line.
We postpone discussion of how vectors might come to be disposed about a line in the co-planar way indicated here, noting now only that, if the vectors are not co-planar, but skew instead, then neither one of the joins of the vector tips cuts the turning line in a point—there is no T, and no vTp.