The Detection of Absolutes (3)

Some unanswered questions

1. How are curves made by strictly projective methods?
2. Can they be made continuous?
3. Can such curves have distinct points in or on them?
4. Assuming that they can
   • be projectively made
   • have distinct points in or on them
   • be continuous,
   is there a distance along the curve between two places on it? If so,
5. Can that distance remain invariant under changes of curvature?

In neither the Rotational nor the Translational case can one, by strictly projective methods, exactly divide an interval into N sub-intervals, where N is any odd integer equal to or greater than 3.

Translational and Rotational step-measures may be made
by strictly projective transformation, independently,
as in the sketch below,

↓but, per items 1 to 5 above right, they are not easily matched.↓

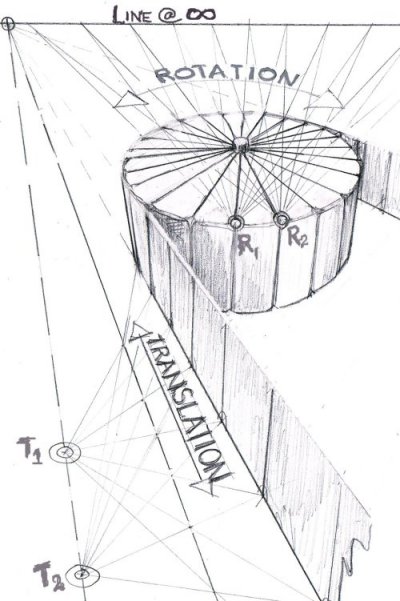
• Despite the seemingly equiangular division of the ‘drum’, depicted above, it is not necessarily exact
• “Measure marks” on ‘drum’ and ‘belt’ could co-incide (i.e., they could be mutually incident), but cannot be made co-incident projectively.

Other examples follow below of a kind essentially similar to the foregoing:

A real rope (such as might tether a real goat) is assumed to be, for all practical purposes, of constant length whether coiled or uncoiled (some might say that this is, “self-evident.” I say it is empirical, and hypothetical.)

A haberdasher's cloth tape-measure is taken to preserve calibration whether pulled taut, or wrapped. (Again some say this is, “self-evident”, and again I say it is empirical and hypothetical.)

And this is the question (item 5, top right)
common to all such examples –

Do strictly-projectively-made curves (given that such things altogether exist) preserve length as belts, ropes and tape-measures appear to do?

Re item 5, above, a pen is expected to deliver ink at a fairly constant rate of so-many litres per unit distance of travel on the written surface, whether or not its path is curved.

Can projective pens do this?

Are there projective pens in sober reality, as opposed to fantasy? Can they write "joined up"?

It is hard to see how they could - they would need to dispense dimensionless ink, indefinitely. (This question relates to the production of locii.)

Do projectively-made surfaces preserve measure like tape-measures and ropes seem to do?

As to that, just how are surfaces projectively made? Note that, if this can be managed at all, it cannot be managed quantitatively, only "incidentally"—that is, via the axioms of elementary incidence, and these alone.

Can there be a projectively-built “printer's cylinder”?

How can such curves or surfaces be made - purely projectively - to roll on each other without slipping? In other words, can there be a projective evolute —

“Evolute: a curve that is the locus of the centers of curvature of another curve (its involute).”

There are geometric involutes, but, unlike the above, they are not metrically defined.

Are projectively-made curves continuous enough to completely support infinitesimals and differentials of the Newtonian/Leibnitzian kind?