Perspective

IFF (‘IF and only iF’) the three conics (here red, green and blue) enclosed in the “box” above and tangent to its faces are all “circles in absolute/physical reality”, and IFF they have a “common centre”, then the box is a cube, and each of the three planes of the circles stands at right angles to the other two.

Notice that, purely projectively, the common centre is the common (degenerate) pole of the three lines (here, the red, green and blue “dot-dash” lines) in which the three conics’ planes cut a fourth plane, and these three lines are the three polars in that plane of this degenerate pole.

These projective things are the case (by the axioms of elementary incidence), whether or not the conics are “absolute” circles, and whether or not the fourth plane is “absolutely” at infinity.

So, IFF the fourth plane lies at infinity, the three polar lines in it meet by twos in the three vanishing points of the three pairs of parallel cuboid faces.

IFF the conics are absolute circles, then the cuboid is a regular cube, and the circles are sections through the centre of the same absolute sphere, so the thing is isotropic, and we appear to have the basis of a co-ordinate system, in perspective.

I stress again that all these things about absolutes and infinity
are purely empirical, and observed,
and so are hypothetical.

I reiterate that they do not arise from elementary incidence,
and are not required by elementary incidence.

*Seeing uses Local Central Projection.* Perspective *Thinking uses Central Projection from Infinity.*		Please enable Java for an interactive construction (with Cinderella).
Please enable Java for an interactive construction (with Cinderella). You may left click and drag the blue dashed line to swing the building around one corner. The roof apex here is in a vertical plane that exactly bisects the building longitudinally.		For “Accurate” Perspective – If the conics “on the ground” in the scene on the left are, “in objective, physical fact”, concentric circles, with radii r, 2r, 3r, ... , and the bold blue line with the “vanishing” points VP₁ and VP₂ *is at the infinite, and is the polar of the common centre of the circles, these points are conjugates in involution, and, if the third vanishing point, VP₃,“below” the glass house also lies in the infinite, the following metric statements* are held to be correct – adjacent walls of this “glass house” are exactly at right angles to each other opposite walls are parallel - that is, at an angle of exactly zero to each other adjacent ‘wall-dividers’ are equidistant from, and parallel to, each other the crests of the four walls are parallel to their foundations the ratio of the length of a long wall to that of a short is exactly 7 to 6 the two, sloping parts of the roof are identical (congruent). 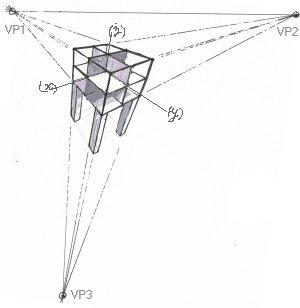A fanciful Cartesian Co-ordinate system — on legs!
Making a Scene Perspective derives from the Axioms of Incidence but only when taken together with two, non-axiomatic things. These are – The notion of special (“ideal”) places (points) at infinity The notion of absolute size ^{[ * ]}. Only the metric statements require these extra items. Geometry itself neither needs nor recognises them, simply because they are not elements, and are not axioms of elementary incidence. This is crucial. To help make this clear, the Three True Elements and most of their Incidences are depicted alongside – and are made visible, incidentally, by endowing the participating elements with physical properties they certainly could not possess!	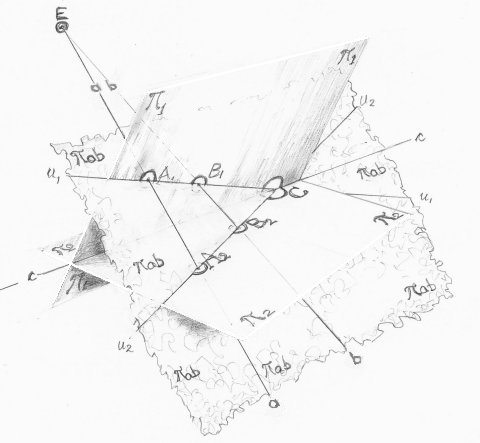Just Elementary Incidence Formally Any two lines, such as a and b, in a point, such as E, have a plane in common, in this case π_ab, which plane is also common to lines u₁ and u₂, So we have three planes, π₁, π₂ and π_ab Their point-in-common is C, which lies in the line c, common to π₁ and π₂.
The General Picture Please enable Java for an interactive construction (with Cinderella). IFF (‘IF and only iF’) the three conics (here red, green and blue) enclosed in the “box” above and tangent to its faces are all “circles in absolute/physical reality”, and IFF they have a “common centre”, then the box is a cube, and each of the three planes of the circles stands at right angles to the other two. Notice that, purely projectively, the common centre is the common (degenerate) pole of the three lines (here, the red, green and blue “dot-dash” lines) in which the three conics’ planes cut a fourth plane, and these three lines are the three polars in that plane of this degenerate pole. These projective things are the case (by the axioms of elementary incidence), whether or not the conics are “absolute” circles, and whether or not the fourth plane is “absolutely” at infinity. So, IFF the fourth plane lies at infinity, the three polar lines in it meet by twos in the three vanishing points of the three pairs of parallel cuboid faces. IFF the conics are absolute circles, then the cuboid is a regular cube, and the circles are sections through the centre of the same absolute sphere, so the thing is isotropic, and we appear to have the basis of a co-ordinate system, in perspective. I stress again that all these things about absolutes and infinity are purely empirical, and observed, and so are hypothetical. I reiterate that they do not arise from elementary incidence, and are not required by elementary incidence.

Perspective

For “Accurate” Perspective –

Making a Scene

Perspective derives from the Axioms of Incidence but only when taken together with two, non-axiomatic things. These are –

Just Elementary Incidence

The General Picture

Perspective derives from the Axioms of Incidence
but only when taken together with
two, non-axiomatic things.
These are –