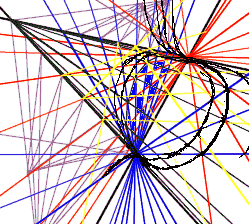
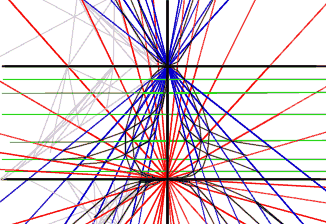
The path curves we have so far seen have been confined to a triangular portion of the plane. It is clear that the confinement of the curve is due to the confined nature of the guiding measures for the line-pair (actually, the line-triple) that define that curve: a measure started in a segment of a line remains in that segment.
Involution, and the Conjugacy derived from it, are the keys to running identical measures in both segments of a line, and we can apply them now to the sides of our invariant triangle—which sides, as we are aware, do not stop at the vertices, because they are lines, and a line does not have "ends" in its list of properties!
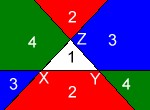
figure I
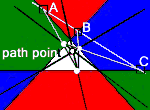
figure II

Path points lie at the intersections of 'fine' red, gold and blue lines. There are three pairs of conjugate path points:
- (1, 2)
- (1, 3)
- (1, 4)
Path point 1 is common to them all.
In fact, a triangle covers its entire plane, and divides it into four, triangular regions. The coloured regions traverse the line at infinity: here, (figure I) the white region does not. Thus the line-segments in which now we wish to install measures divide colour from colour, but not colour from white.
The involutions are already embedded. To reveal them, we "put in the diagonals", so to speak, of a path point in the white region (figure II). The three cuts, A, B and C, by these diagonals of the triangle's sides must, by Desargue's Theorem, lie in a straight line, and they are, in fact, the conjugate points we seek in the sides. Lines from opposite vertices to them are the stepping lines we need to find the conjugate path points.
We are now in a position to populate the entire plane with path curves!