Two Skew Intervals
Here we have the two perspectivities, centred on
S and P, on different planes
incident in the line of the black interval, as before,
with the two intervals
under comparison with the black skew to each other.
As we will see in due course, this
one opens a major route to
the imaginary, and to projective comparison of rotations.
We show the perspectivities as if draped on the slopes of a roof, with the line of incidence of their planes on the apex. You may notice that the two perspectivities and the line PS form a tetrahedron, with its four planes. Two of these are the roof slopes. Where are the other two?
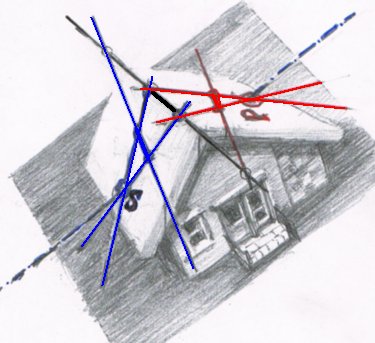
Do note that if the red and blue intervals become coplanar, not skew as they are here, their lines must meet in a single point in the line of the black interval, not in two as they now do, and the entire configuration must degenerate into the Desargues Mode. Again as we shall see, this has some rather important implications.