Desargues Mode
When the two perspectivities are not co-planar, and the lines bearing our three equivalent intervals are all taken though a single point, as above, we obtain the famous Desargues configuration, which concerns a particular relationship between two triangles, here Δ TUV and Δ T'U'V'.
The truth of Desargues Theorem is trivially obvious if the triangles
Δ TUV and
Δ
T'U'V' are in different planes, as they are here: the lines connecting
the vertices through C are just our three interval-lines. The Δ's are ‘shadows’ of each
other with respect to ‘source’ C. Corresponding triangle lines
(mutual ‘shadows’) must
meet in points in the line of incidence of the triangles' planes. Point C
is called the “perspector”, and the line of incidence of the two
planes, through P, S and D, is called the “perspectrix”.
Desargues: the deep base → Five Planes
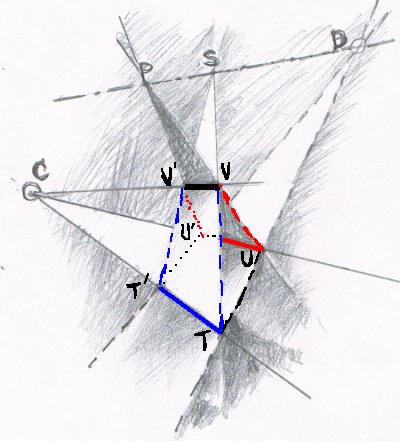
However, in truth, the illustrations here have two triangles actually in the same plane - which is that of the screen: it is a set of ‘shadows’ (projections), from a point (‘centre’), of spatially distributed elements. This coplanar case is the degenerate case. Given coplanar cases such as these, we are always able to (re)generate a spatial, non-degenerate construction, and a centre, that together give rise to it.