. Difference ←Tutorial page                  ← Related page                  ← Comparisons 5: Iteration                  Comparisons 7: Replication →                  Formal proof →

Projective Mismatch

Suppose we come across a line or lines, with some intervals already set out on them, like the dog-walker is doing in the rather whimsical sketch below, entitled, "Star Wars in Somerset". Are all these intervals, between A, and B, and C, and so on,  projectively equivalent (identical)? This is simply to ask, “Are they projectively distinguishable?”  How may we find out?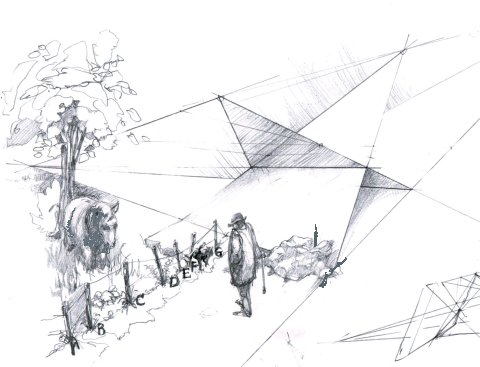

a_no_same 		Well, we know that a linear, or interpunctual, interval, such as that from A to B, is identical with another if they both participate in a perspectivity – or, if each is in its own perspectivity with a third, common interval, as components of a projectivity – so all we need do is see whether or not we can set these structures up for our adventitious intervals.  If we can, the intervals in question must be indistinguishable.  If we can't, they must be different, inequivalent, inidentical, and altogether fail to match ..

The simplest approach is to take two of these intervals as the basis of the projective ruler that we will use to assess all the others – by elementary incidence, of course, as always.  In other words, we first define our standard reference.

This is easily done:  Take two contiguous, daisy-chained intervals, AB, and BC, say.  Draw lines from their common end, B, through the end-points, say M and N, of a black interval nearby.  Then, draw first from A through M to meet BN in P, and then from C through N to meet BM in S.  We get perspectivities forming projectivities that make all these intervals equivalent.  We have now only to test whether the remaining intervals form perspectivities with interval PS via points on the black line.. 		And we see that interval EF certainly does not do that, while interval FG perhaps does. So EF is not identical to PS, or to AB, or to BC, but FG may be.



The others, CD and DE, could be similarly tested.

We note again that our choices lead to exactly two, so-called ‘invariant points’, X and Y, which may be said to ‘end’ the entire ruler, just as end-points end the intervals of which the ruler is fashioned.
←Tutorial page                  ← Related page                  ← Comparisons 5: Iteration                  Comparisons 7: Replication →                  Formal proof →